







| The Quantum World - Atoms |
Below are some models of some atoms. These models illustrate various shapes adopted by the hydrogen atom - the shape
adopted depending upon the energy of the atom.
(Tech Note: These models are not exact mathematical plots, but are constructed from constructive solid geometry in Pov
Ray). For some exact mathematical plots click here.
adopted depending upon the energy of the atom.
(Tech Note: These models are not exact mathematical plots, but are constructed from constructive solid geometry in Pov
Ray). For some exact mathematical plots click here.
100
210
310
311
210
321
322
430
431
What is an atom?
Familiar and 'ordinary' matter, like the wood in a desk, the meat on your bones, the stones in your garden, as well as the water in
your tank and the air in your room, are all made up of atoms. Atoms are particles. Think of smoke, we often talk about 'particles' of
smoke, meaning the little black bits of soot and ash that make up smoke - we say that the smoke is made up of particles. So too,
matter is made up of particles called atoms. Indeed, the particles of smoke are really large clumps of atoms, so particles can be
made up of smaller particles. The atoms in the pictures above are actually atoms of hydrogen gas. Hydrogen gas has the simplest
atoms that there are. The atoms of hydrogen gas are too small to be seen by the naked eye, or even by a standard microscope (in
fact they are so small that they cannot be seen by light at all and so require special methods of detection). The exact size of an
atom varies and depends how it is measured, but a hydrogen atom is about 0.1 nanometres in diameter (a nanometre is one
millionth of a millimetre). Thus, to span a length of one millimetre (one 25th of an inch) requires something like 10 million hydrogen
atoms side-by-side in a line! So you see, atoms really are incredibly tiny.
Atoms make up elements
Hydrogen is what we call an element. Iron, carbon, oxygen, sulphur and uranium are all elements. There are currently something
like 112 known elements, though the exact number is under dispute because some of them are so unstable that their atoms
collapse and decay almost as soon as you detect them. Fortunately, however, enough are stable to give the 92 elements found in
nature. An iron rod weighing one kilogram (2.2 pounds) contains roughly 10 000 000 000 000 000 000 000 000 atoms of iron (1 x
10^25 or 1E+25 atoms).
Atoms make molecules
Atoms can stick or bond together in certain permissible combinations to form groups of atoms called molecules. In the element
sulphur, atoms group together to form rings of 8 atoms. These rings group together to form a stone of sulphur, such as what you
might find in a rock shop. These rings, and other similar groups of atoms, are called molecules. Atoms of different elements may
bond together to form molecules called compounds, because they are compounded from more than one type of atom. Water is
made up of H2O molecules - molecules made up of two hydrogen (H) atoms and one oxygen (O) atom bonded together.
Atoms are made up of vibrations or waves
Think of a water wave on the sea. You would be right in thinking that a wave is a repeating or periodic oscillation or vibration, in this
case of the water's surface. Sound waves are vibrations that travel through the air (or water or through a solid wall). Light waves
are electromagnetic vibrations. In fact, just about everything that you can think of is actually made up of vibrations. Atoms are no
exception, so we need to say a little about waves first. Consider a simple wave, either a water wave or a vibration on a string or
slinky spring. In profile it might look something like the diagram below:
Familiar and 'ordinary' matter, like the wood in a desk, the meat on your bones, the stones in your garden, as well as the water in
your tank and the air in your room, are all made up of atoms. Atoms are particles. Think of smoke, we often talk about 'particles' of
smoke, meaning the little black bits of soot and ash that make up smoke - we say that the smoke is made up of particles. So too,
matter is made up of particles called atoms. Indeed, the particles of smoke are really large clumps of atoms, so particles can be
made up of smaller particles. The atoms in the pictures above are actually atoms of hydrogen gas. Hydrogen gas has the simplest
atoms that there are. The atoms of hydrogen gas are too small to be seen by the naked eye, or even by a standard microscope (in
fact they are so small that they cannot be seen by light at all and so require special methods of detection). The exact size of an
atom varies and depends how it is measured, but a hydrogen atom is about 0.1 nanometres in diameter (a nanometre is one
millionth of a millimetre). Thus, to span a length of one millimetre (one 25th of an inch) requires something like 10 million hydrogen
atoms side-by-side in a line! So you see, atoms really are incredibly tiny.
Atoms make up elements
Hydrogen is what we call an element. Iron, carbon, oxygen, sulphur and uranium are all elements. There are currently something
like 112 known elements, though the exact number is under dispute because some of them are so unstable that their atoms
collapse and decay almost as soon as you detect them. Fortunately, however, enough are stable to give the 92 elements found in
nature. An iron rod weighing one kilogram (2.2 pounds) contains roughly 10 000 000 000 000 000 000 000 000 atoms of iron (1 x
10^25 or 1E+25 atoms).
Atoms make molecules
Atoms can stick or bond together in certain permissible combinations to form groups of atoms called molecules. In the element
sulphur, atoms group together to form rings of 8 atoms. These rings group together to form a stone of sulphur, such as what you
might find in a rock shop. These rings, and other similar groups of atoms, are called molecules. Atoms of different elements may
bond together to form molecules called compounds, because they are compounded from more than one type of atom. Water is
made up of H2O molecules - molecules made up of two hydrogen (H) atoms and one oxygen (O) atom bonded together.
Atoms are made up of vibrations or waves
Think of a water wave on the sea. You would be right in thinking that a wave is a repeating or periodic oscillation or vibration, in this
case of the water's surface. Sound waves are vibrations that travel through the air (or water or through a solid wall). Light waves
are electromagnetic vibrations. In fact, just about everything that you can think of is actually made up of vibrations. Atoms are no
exception, so we need to say a little about waves first. Consider a simple wave, either a water wave or a vibration on a string or
slinky spring. In profile it might look something like the diagram below:
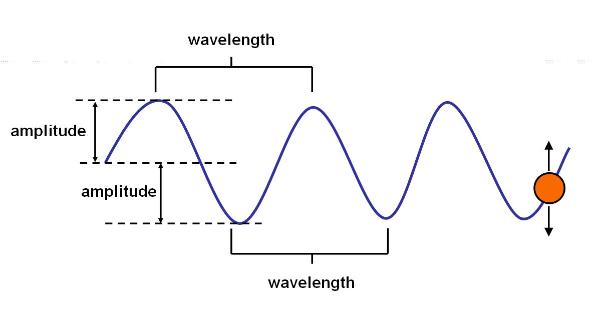
As the water moves up and down, we have peaks (the crests of the wave) and troughs (the dips). We define the wavelength to
be the distance between adjacent peaks (or equivalently between adjacent troughs or between any two equivalent points along
the wave). (Note this is not the length of the wave in common terms). For a water wave we may have a wavelength of 10 metres
say, though the wave may spread for a thousand kilometres across the Atlantic Ocean. Half the height of the wave we define as
the amplitude. We chose half the height because this is the height of the wave above the undisturbed water surface, since the
peaks move water up and the troughs move water just as far, but downwards. We can see our little orange buoy bobbing up and
down, but note that it doesn't move sideways much, since the water moves up and down but does not travel along with the wave
from say left to right. If we count how often our buoy bobs up in say one minute, then we get the frequency of the wave - how often
a crest passes. For a water wave we might get a frequency of 12 wave crests a minute (one crest passing the buoy every 5
seconds). If the wavelength is 10 m then the wave speed is (10 x 12 = 120) 120 metres per minute (or 2 metres per second or
7.2 kph). One can also speak of the wave period as the period of time between two wave crests, in this case 5 seconds.
(Tech note: Actually the water moves in circular or elliptical orbits, and there is, contrary to what most physics and maths
textbooks say, also some sideways displacement of the water, especially when the waves are deep, since the equations of motion
then become nonlinear).
Our water wave may move along the water though, from say east to west, even if the water does not move sideways with it - the
disturbance spreads across the water, not the water molecules themselves. Such a wave is called a travelling wave. However,
waves can do odd things, like reflect off solid obstacles (light reflects off mirrors, so water waves reflect off harbour walls). The
weird thing about waves is that they interact with one another when they occupy the same space, so the reflected wave interacts
with the incoming wave as the two overlap. If the peak of the reflected wave coincides with the troughs of the incoming wave (and
so the troughs of the reflected wave interact with the peaks of the incoming wave) then what happens?
The waves will cancel out! If, however, the peaks coincide with one another and the troughs coincide then we get a single wave
with twice the amplitude of the incoming wave. This process of waves interacting with each other is called interference. When
they cancel each other out, we have destructive interference, and when they add to give a deeper wave, we have
constructive interference.
Inside the harbour walls things could get complicated, with so many waves bouncing around off walls. Not all of these waves will
have the same wavelength either, however, the water does not chop and churn all over the place (except in a storm!). This is
because most of the waves get cancelled out by each other. Only waves of certain wavelengths survive by constructive
interference, the majority are destroyed. We actually end up with a few waves of certain wavelengths that bounce back and forth
and end up going nowhere - these are called stationary waves.
Ok we are nearly ready to understand the shapes of our atoms, but first we need to say a bit more about waves, using music as
our example.
be the distance between adjacent peaks (or equivalently between adjacent troughs or between any two equivalent points along
the wave). (Note this is not the length of the wave in common terms). For a water wave we may have a wavelength of 10 metres
say, though the wave may spread for a thousand kilometres across the Atlantic Ocean. Half the height of the wave we define as
the amplitude. We chose half the height because this is the height of the wave above the undisturbed water surface, since the
peaks move water up and the troughs move water just as far, but downwards. We can see our little orange buoy bobbing up and
down, but note that it doesn't move sideways much, since the water moves up and down but does not travel along with the wave
from say left to right. If we count how often our buoy bobs up in say one minute, then we get the frequency of the wave - how often
a crest passes. For a water wave we might get a frequency of 12 wave crests a minute (one crest passing the buoy every 5
seconds). If the wavelength is 10 m then the wave speed is (10 x 12 = 120) 120 metres per minute (or 2 metres per second or
7.2 kph). One can also speak of the wave period as the period of time between two wave crests, in this case 5 seconds.
(Tech note: Actually the water moves in circular or elliptical orbits, and there is, contrary to what most physics and maths
textbooks say, also some sideways displacement of the water, especially when the waves are deep, since the equations of motion
then become nonlinear).
Our water wave may move along the water though, from say east to west, even if the water does not move sideways with it - the
disturbance spreads across the water, not the water molecules themselves. Such a wave is called a travelling wave. However,
waves can do odd things, like reflect off solid obstacles (light reflects off mirrors, so water waves reflect off harbour walls). The
weird thing about waves is that they interact with one another when they occupy the same space, so the reflected wave interacts
with the incoming wave as the two overlap. If the peak of the reflected wave coincides with the troughs of the incoming wave (and
so the troughs of the reflected wave interact with the peaks of the incoming wave) then what happens?
The waves will cancel out! If, however, the peaks coincide with one another and the troughs coincide then we get a single wave
with twice the amplitude of the incoming wave. This process of waves interacting with each other is called interference. When
they cancel each other out, we have destructive interference, and when they add to give a deeper wave, we have
constructive interference.
Inside the harbour walls things could get complicated, with so many waves bouncing around off walls. Not all of these waves will
have the same wavelength either, however, the water does not chop and churn all over the place (except in a storm!). This is
because most of the waves get cancelled out by each other. Only waves of certain wavelengths survive by constructive
interference, the majority are destroyed. We actually end up with a few waves of certain wavelengths that bounce back and forth
and end up going nowhere - these are called stationary waves.
Ok we are nearly ready to understand the shapes of our atoms, but first we need to say a bit more about waves, using music as
our example.
Click image on left for plots.
Waves and music
The same is true on a guitar string. A guitar string is a string fixed at both ends. When the string is struck, waves travel along the
string, but soon reflect off both the fixed ends, until only certain wavelengths remain. The string causes the guitar to vibrate and
the guitar causes the air to vibrate in a similar manner and we hear a sound based on the waves on the string. Notice we hear a
definite note not a chaotic clatter - this is because only certain wavelengths, called the harmonics remain. Which harmonics
remain depend upon the length of the string and the reflective properties of the material that fixes the ends of the string. Of
course our guitarist can change the effective length of each string by pinning it to the fret board with their fingers, altering the
note of each string as they play. The waves on the string are standing waves which vibrate up and down but do not appear to
move from side to side.
The diagram below shows the first three harmonics for a vibrating string:
The same is true on a guitar string. A guitar string is a string fixed at both ends. When the string is struck, waves travel along the
string, but soon reflect off both the fixed ends, until only certain wavelengths remain. The string causes the guitar to vibrate and
the guitar causes the air to vibrate in a similar manner and we hear a sound based on the waves on the string. Notice we hear a
definite note not a chaotic clatter - this is because only certain wavelengths, called the harmonics remain. Which harmonics
remain depend upon the length of the string and the reflective properties of the material that fixes the ends of the string. Of
course our guitarist can change the effective length of each string by pinning it to the fret board with their fingers, altering the
note of each string as they play. The waves on the string are standing waves which vibrate up and down but do not appear to
move from side to side.
The diagram below shows the first three harmonics for a vibrating string:

Notice that the number of peaks plus the number of troughs gives the harmonic, so the second harmonic has one peak plus one
trough, the 4th harmonic has two peaks and two troughs, etc. Each note will consist of many harmonics, but the lower harmonic
are the most important. The higher harmonics add quality to the note and depend upon the individual instrument. All the
harmonics actually contain a whole number of half wavelengths. The fundamental is the first harmonic and is just half a
wavelength )because it has one peak but no trough). The exact combination of harmonics is unique to each instrument or
human voice, and is called the timbre (quality) of the sound.
Even if all the strings on a guitar are kept at the same length they all make different notes, why is this? The harmonics are the
same, since the fundamental wavelength is just twice the string length. What differs between strings is not the wavelengths of
the harmonics, but their frequencies. The frequency of a wave depends both upon the wavelength and the speed of the wave
(note standing waves still have speed even though they appear stationary!). The wave speed depends upon the tension and
weight (density) of the string. A lighter or a tenser string produces a higher frequency., A higher pitched string has a higher
wave speed and produces notes of a higher frequency and hence of a higher pitch. Shorter strings also have shorter
wavelengths for their harmonics and so higher frequencies and higher pitches. Thus a violin produces higher pitched notes than
the double bass.
From strings to atoms
Nearly there! Think now of a drum. A drum vibrates when struck just like a string. A drum also vibrates with certain frequencies
or harmonics, but this time the vibrations are those of a sheet (we have a 2D wave). Think of a bell. A bell also vibrates
according to its harmonic frequencies, with larger bells producing deeper sounds, but this time we have a 3D wave.
Atoms are rather like spherical bells that vibrate. As atoms vibrate they change shape. Being tiny objects they adopt shapes that
differ dramatically from one another according to the harmonic or mode of their vibration. Sometimes they vibrate with several
harmonics, but they often have a preference to vibrate with only one harmonic, called the mode of vibration. The pictures on
the previous page showed several hydrogen atoms, in fact they could all be the same hydrogen atom at different times. Each
shape is a standing wave as the atom vibrates, so the first one, labelled 100 is spherical, as if the atom is not vibrating at all but
is at rest. Other modes give very different shapes!
The mode of vibration of an atom depends upon its energy
The more energy we give to an atom (e.g. by hitting it with light or a laser beam) the higher the mode of the vibration (the higher
the frequency of its harmonic). Each vibration mode is assigned three numbers (called quantum numbers), e.g. 100, 321, etc.
The first number is the energy of the vibration mode, so 200 is more energetic than 100 and 311 is more energetic than 200.
This is called the principal quantum number (n) and is given the symbol n. The second number is due to something called
angular momentum and is called the angular momentum quantum number (l). We talk of momentum when an object is
moving, the faster it moves or the heavier it is, the more momentum it has and the harder it is (the more force required) to stop
it. This is ordinary linear momentum, when an object moves in a straight line. However, objects that spin also have angular
momentum, as does pirouetting ice skater. An atom may have angular momentum (though this is somewhat different to the
angular momentum of an ice skater). The 2nd and 3rd numbers give us the precise shape of the atom, if they are both zero, as
in 100, 200, 300 , ... , then the atom is spherical. If the 2nd quantum number is equal to 1 or greater, then the atom has a
dumb-bell, torus (doughnut) or more complicated shape. The third number has to do with the shape of the atom in a magnetic
field, and is called the magnetic quantum number (m).
Where do the quantum numbers come from?
The quantum numbers and the shapes of the atom are obtained by solving what is known as Schrodinger's wave equation.
Schrodinger proposed this equation based upon our knowledge of maths and physics. This equation has more than one
solution, one for each possible value of the three quantum numbers. Each such solution is called a wave function and the wave
function tells us most of what we can know about the shape and behaviour of the atom (I say 'most' because there are various
extra corrections for things we call spin, relativity, internal and external magnetic and electric fields and quantum
electrodynamics). Actual observations and measurements verify the Schrodinger wave equation, so we can be reasonably
confident that our physical model of the atom is correct. In fact, quantum mechanics, the branch of science that uses the
Schrodinger equation amongst many others, can describe the hydrogen atom very precisely indeed! This is a major triumph for
science. I am not going to give the equation or its solutions here - to explain these would take a whole book, and plenty of
people have already written such books! The key point is, that these mathematical equations allow us not only to accurately
describe the shapes of atoms, but also to accurately and reliably predict their behaviour - so we can make predictions and then
carry out experiments to test those predictions, and refine the theory if need be and learn new physics.
What does 'quantum' mean?
Notice that the atom can not vibrate in any old manner, just as our guitar string cannot, rather it can only vibrate in certain ways
(the harmonics). Since each harmonic has a certain well defined frequency and so energy, this means that the atom cannot
possess any old energy, but only certain values of energy. The energy is divided into a series of discrete 'energy levels' and we
say that the energy is quantised (made up of packets of a certain size). It turns out that many properties of many things in
physics are quantised according to the same principles (almost everything is made up of vibrations). The study of these
quantised processes is called quantum mechanics or quantum physics.
What exactly is an atom made of - what is actually vibrating?
So far we have talked about atoms as tiny bells that vibrate. This is an over-simplistic model. The real world of the atom is very
very different from the large-scale world that we are familiar with from everyday experience. Atoms can do odd things, like
behave as if they are in two or more places at once, or teleport from one place to another in an instance. The world
of the atom is simply different!
Atoms are made up of smaller particles, called electrons, protons and neutrons. The protons and neutrons cluster together
in the centre of the atom, forming the nucleus. The electrons form the vibrating shells that we have seen pictures of, around the
nucleus. Hydrogen atoms only have one electron and one proton (and usually no neutrons) but other atoms contain many of
these particles. The nucleus contains almost all of the atom's mass, but is very tiny indeed - about one hundred thousandth the
diameter of the atom (1 x 10^-15 metres diameter). Being so small, but containing most of the atom's mass, the nucleus is
extremely dense! People used to think of the atom like the Solar System, with the electrons representing the planets orbiting the
nucleus which represented the Sun. This is not correct, however. The model that we have discussed is far more correct, but
requires a deeper understanding. The problem is a phenomenon called wave-particle duality.
We have talked about atoms as particles, like tiny solid balls (or bells). However, an atom can also behave like a wave. We saw
that an atom is made up of waves, but it can also be a wave! This confuses most people (if not everybody!) but it shouldn't
really, because almost everything is made up of vibrations anyway. What one takes for granted to be a solid table, is made up of
atoms which vibrate and the electrons inside the atoms vibrate - the whole table consists of energy vibrations. So atoms, and
indeed electrons, protons, neutrons, in fact any particle, can also be a wave, this is the phenomenon of wave-particle duality.
Really, particles are waves that sometimes resemble particles.
When you lean against the table it feels 'solid' because the electrons in the atoms in your body are repelled by the electrons in
the atoms of the table. This is because electrons carry negative electric charge and like charges repel. The protons in
the nucleus carry positive electric charge and opposite charges attract, so the electrons are attracted to the nucleus and
tend to stay close to it. Thus we can imagine electrons forming negatively charged shells that surround the positively charged
central nucleus. The reason that electric charges repel or attract one another is that they exchange photons. Photons are
particles of light, so the electrons and protons exchange a kind of light, however, it is not the kind of light we can see, because
the photons exist for a period of time that makes it impossible in principle to ever detect them. It is as if these photons never
exist, and so they are called virtual photons. The light that you see consists of photons of the non-virtual variety! So, in a
sense an atom is made up of waves (particles called electrons, protons and neutrons) bound together by (virtual) light, which is
itself a wave. Thus, waves hold the waves together!
So what vibrates? Well, everything just about, but the electrons that form shells (or orbitals) around the nucleus are vibrating to
give the atoms their shapes. However, this is not quite correct! Atoms are far more mysterious. If you measure the electron in a
hydrogen atom, to see how it is vibrating, then you will instead find that it exists as a single discrete particle at some point near
to the nucleus (or sometimes inside it and sometimes far away), so what happened - is our model wrong? No, not exactly, what is
vibrating is not the electron itself, as such, but the wave function that describes its properties, such as its position and speed.
However, that is not all, the electron actually does not have any position or speed until we measure it! (Or it may have any,
every or no position!).
trough, the 4th harmonic has two peaks and two troughs, etc. Each note will consist of many harmonics, but the lower harmonic
are the most important. The higher harmonics add quality to the note and depend upon the individual instrument. All the
harmonics actually contain a whole number of half wavelengths. The fundamental is the first harmonic and is just half a
wavelength )because it has one peak but no trough). The exact combination of harmonics is unique to each instrument or
human voice, and is called the timbre (quality) of the sound.
Even if all the strings on a guitar are kept at the same length they all make different notes, why is this? The harmonics are the
same, since the fundamental wavelength is just twice the string length. What differs between strings is not the wavelengths of
the harmonics, but their frequencies. The frequency of a wave depends both upon the wavelength and the speed of the wave
(note standing waves still have speed even though they appear stationary!). The wave speed depends upon the tension and
weight (density) of the string. A lighter or a tenser string produces a higher frequency., A higher pitched string has a higher
wave speed and produces notes of a higher frequency and hence of a higher pitch. Shorter strings also have shorter
wavelengths for their harmonics and so higher frequencies and higher pitches. Thus a violin produces higher pitched notes than
the double bass.
From strings to atoms
Nearly there! Think now of a drum. A drum vibrates when struck just like a string. A drum also vibrates with certain frequencies
or harmonics, but this time the vibrations are those of a sheet (we have a 2D wave). Think of a bell. A bell also vibrates
according to its harmonic frequencies, with larger bells producing deeper sounds, but this time we have a 3D wave.
Atoms are rather like spherical bells that vibrate. As atoms vibrate they change shape. Being tiny objects they adopt shapes that
differ dramatically from one another according to the harmonic or mode of their vibration. Sometimes they vibrate with several
harmonics, but they often have a preference to vibrate with only one harmonic, called the mode of vibration. The pictures on
the previous page showed several hydrogen atoms, in fact they could all be the same hydrogen atom at different times. Each
shape is a standing wave as the atom vibrates, so the first one, labelled 100 is spherical, as if the atom is not vibrating at all but
is at rest. Other modes give very different shapes!
The mode of vibration of an atom depends upon its energy
The more energy we give to an atom (e.g. by hitting it with light or a laser beam) the higher the mode of the vibration (the higher
the frequency of its harmonic). Each vibration mode is assigned three numbers (called quantum numbers), e.g. 100, 321, etc.
The first number is the energy of the vibration mode, so 200 is more energetic than 100 and 311 is more energetic than 200.
This is called the principal quantum number (n) and is given the symbol n. The second number is due to something called
angular momentum and is called the angular momentum quantum number (l). We talk of momentum when an object is
moving, the faster it moves or the heavier it is, the more momentum it has and the harder it is (the more force required) to stop
it. This is ordinary linear momentum, when an object moves in a straight line. However, objects that spin also have angular
momentum, as does pirouetting ice skater. An atom may have angular momentum (though this is somewhat different to the
angular momentum of an ice skater). The 2nd and 3rd numbers give us the precise shape of the atom, if they are both zero, as
in 100, 200, 300 , ... , then the atom is spherical. If the 2nd quantum number is equal to 1 or greater, then the atom has a
dumb-bell, torus (doughnut) or more complicated shape. The third number has to do with the shape of the atom in a magnetic
field, and is called the magnetic quantum number (m).
Where do the quantum numbers come from?
The quantum numbers and the shapes of the atom are obtained by solving what is known as Schrodinger's wave equation.
Schrodinger proposed this equation based upon our knowledge of maths and physics. This equation has more than one
solution, one for each possible value of the three quantum numbers. Each such solution is called a wave function and the wave
function tells us most of what we can know about the shape and behaviour of the atom (I say 'most' because there are various
extra corrections for things we call spin, relativity, internal and external magnetic and electric fields and quantum
electrodynamics). Actual observations and measurements verify the Schrodinger wave equation, so we can be reasonably
confident that our physical model of the atom is correct. In fact, quantum mechanics, the branch of science that uses the
Schrodinger equation amongst many others, can describe the hydrogen atom very precisely indeed! This is a major triumph for
science. I am not going to give the equation or its solutions here - to explain these would take a whole book, and plenty of
people have already written such books! The key point is, that these mathematical equations allow us not only to accurately
describe the shapes of atoms, but also to accurately and reliably predict their behaviour - so we can make predictions and then
carry out experiments to test those predictions, and refine the theory if need be and learn new physics.
What does 'quantum' mean?
Notice that the atom can not vibrate in any old manner, just as our guitar string cannot, rather it can only vibrate in certain ways
(the harmonics). Since each harmonic has a certain well defined frequency and so energy, this means that the atom cannot
possess any old energy, but only certain values of energy. The energy is divided into a series of discrete 'energy levels' and we
say that the energy is quantised (made up of packets of a certain size). It turns out that many properties of many things in
physics are quantised according to the same principles (almost everything is made up of vibrations). The study of these
quantised processes is called quantum mechanics or quantum physics.
What exactly is an atom made of - what is actually vibrating?
So far we have talked about atoms as tiny bells that vibrate. This is an over-simplistic model. The real world of the atom is very
very different from the large-scale world that we are familiar with from everyday experience. Atoms can do odd things, like
behave as if they are in two or more places at once, or teleport from one place to another in an instance. The world
of the atom is simply different!
Atoms are made up of smaller particles, called electrons, protons and neutrons. The protons and neutrons cluster together
in the centre of the atom, forming the nucleus. The electrons form the vibrating shells that we have seen pictures of, around the
nucleus. Hydrogen atoms only have one electron and one proton (and usually no neutrons) but other atoms contain many of
these particles. The nucleus contains almost all of the atom's mass, but is very tiny indeed - about one hundred thousandth the
diameter of the atom (1 x 10^-15 metres diameter). Being so small, but containing most of the atom's mass, the nucleus is
extremely dense! People used to think of the atom like the Solar System, with the electrons representing the planets orbiting the
nucleus which represented the Sun. This is not correct, however. The model that we have discussed is far more correct, but
requires a deeper understanding. The problem is a phenomenon called wave-particle duality.
We have talked about atoms as particles, like tiny solid balls (or bells). However, an atom can also behave like a wave. We saw
that an atom is made up of waves, but it can also be a wave! This confuses most people (if not everybody!) but it shouldn't
really, because almost everything is made up of vibrations anyway. What one takes for granted to be a solid table, is made up of
atoms which vibrate and the electrons inside the atoms vibrate - the whole table consists of energy vibrations. So atoms, and
indeed electrons, protons, neutrons, in fact any particle, can also be a wave, this is the phenomenon of wave-particle duality.
Really, particles are waves that sometimes resemble particles.
When you lean against the table it feels 'solid' because the electrons in the atoms in your body are repelled by the electrons in
the atoms of the table. This is because electrons carry negative electric charge and like charges repel. The protons in
the nucleus carry positive electric charge and opposite charges attract, so the electrons are attracted to the nucleus and
tend to stay close to it. Thus we can imagine electrons forming negatively charged shells that surround the positively charged
central nucleus. The reason that electric charges repel or attract one another is that they exchange photons. Photons are
particles of light, so the electrons and protons exchange a kind of light, however, it is not the kind of light we can see, because
the photons exist for a period of time that makes it impossible in principle to ever detect them. It is as if these photons never
exist, and so they are called virtual photons. The light that you see consists of photons of the non-virtual variety! So, in a
sense an atom is made up of waves (particles called electrons, protons and neutrons) bound together by (virtual) light, which is
itself a wave. Thus, waves hold the waves together!
So what vibrates? Well, everything just about, but the electrons that form shells (or orbitals) around the nucleus are vibrating to
give the atoms their shapes. However, this is not quite correct! Atoms are far more mysterious. If you measure the electron in a
hydrogen atom, to see how it is vibrating, then you will instead find that it exists as a single discrete particle at some point near
to the nucleus (or sometimes inside it and sometimes far away), so what happened - is our model wrong? No, not exactly, what is
vibrating is not the electron itself, as such, but the wave function that describes its properties, such as its position and speed.
However, that is not all, the electron actually does not have any position or speed until we measure it! (Or it may have any,
every or no position!).
So, isn't it confusing so far?
Well, at least it is mysterious. Physicists have a famous saying: If you think that you understand quantum mechanics then you
don't! Thus, if you are not understanding so far, then you are in good company! The trouble is, we only feel as if we
understand something when we can reduce or relate it to events in our everyday life. What we call 'common sense' is actually
a delusion! When a young child grows they know very little about the world and they may believe in Father Christmas and
tooth fairies. They are not silly to think like this, the brain evolved to be plastic so that it can learn about the world no matter
what world it finds itself in. It is not wise to make assumptions! When we think that we learn something from experience, the
problem is we have only experienced a very limited part of the Universe and when we try to understand the rest, we cannot!
Our experiences are just approximations - what we think we know is just an approximate model of the world which our brains
have constructed. The world of the very small is simply different! If we lived in that world every day, then teleportation,
appearing in several places in once, turning into waves, and so on, would make perfect sense to us! So, relax, don't try too
hard but just enjoy seeing something that you may not have seen before ...
So what are the electrons in atoms up to?
Until we measure the electron's position it has no exact position. Until we measure its speed, it has no exact speed. The
electron (and the atom) is so tiny, that simply by measuring it we disturb it and change it. The exact interpretation is still
controversial, but we shall stick to the most widely accepted one. When nobody is looking the electron spreads itself out a bit -
it can be nowhere but is probably in several different places at once. However, as soon as we detect it, say by hitting it with a
photon, it collapses into a well defined state - it suddenly acquires a definite position and speed. This is just as well, it would be
confusing to find it in several places! Left to its own devices, it will eventually spread itself again. However, it is not equally likely
to be found everywhere or anywhere because it is attracted to the nucleus and so it is much more likely to be found within a
certain small distance of the nucleus than a thousand miles away. The wave function tells us the probability that it will be found
in a given place with a given speed and a given angular momentum, etc. In fact the wave function gives us a complete
description of the electron's observable properties. Experiments suggest that the electron cannot have hidden properties that
we cannot observe (though this is not known for definite). The pictures we saw were simply the shells around the nucleus
where the electron is most likely to be found, but these shells are really fuzzy, sometimes it will be very close to the nucleus
(sometimes inside the nucleus if it is allowed in) and sometimes it will be far away from the nucleus, but most of the time it will
be within the shell described by the harmonic.
For example, the spherical 200 orbital might look something like the picture on the left below, rather than the one on the right:
Well, at least it is mysterious. Physicists have a famous saying: If you think that you understand quantum mechanics then you
don't! Thus, if you are not understanding so far, then you are in good company! The trouble is, we only feel as if we
understand something when we can reduce or relate it to events in our everyday life. What we call 'common sense' is actually
a delusion! When a young child grows they know very little about the world and they may believe in Father Christmas and
tooth fairies. They are not silly to think like this, the brain evolved to be plastic so that it can learn about the world no matter
what world it finds itself in. It is not wise to make assumptions! When we think that we learn something from experience, the
problem is we have only experienced a very limited part of the Universe and when we try to understand the rest, we cannot!
Our experiences are just approximations - what we think we know is just an approximate model of the world which our brains
have constructed. The world of the very small is simply different! If we lived in that world every day, then teleportation,
appearing in several places in once, turning into waves, and so on, would make perfect sense to us! So, relax, don't try too
hard but just enjoy seeing something that you may not have seen before ...
So what are the electrons in atoms up to?
Until we measure the electron's position it has no exact position. Until we measure its speed, it has no exact speed. The
electron (and the atom) is so tiny, that simply by measuring it we disturb it and change it. The exact interpretation is still
controversial, but we shall stick to the most widely accepted one. When nobody is looking the electron spreads itself out a bit -
it can be nowhere but is probably in several different places at once. However, as soon as we detect it, say by hitting it with a
photon, it collapses into a well defined state - it suddenly acquires a definite position and speed. This is just as well, it would be
confusing to find it in several places! Left to its own devices, it will eventually spread itself again. However, it is not equally likely
to be found everywhere or anywhere because it is attracted to the nucleus and so it is much more likely to be found within a
certain small distance of the nucleus than a thousand miles away. The wave function tells us the probability that it will be found
in a given place with a given speed and a given angular momentum, etc. In fact the wave function gives us a complete
description of the electron's observable properties. Experiments suggest that the electron cannot have hidden properties that
we cannot observe (though this is not known for definite). The pictures we saw were simply the shells around the nucleus
where the electron is most likely to be found, but these shells are really fuzzy, sometimes it will be very close to the nucleus
(sometimes inside the nucleus if it is allowed in) and sometimes it will be far away from the nucleus, but most of the time it will
be within the shell described by the harmonic.
For example, the spherical 200 orbital might look something like the picture on the left below, rather than the one on the right:
In constructing the one on the right, we chose to consider only a surface of constant probability density, as it is called, which
means a surface within which we will find the electron, say 90% of the time. In section the orbital on the left, now really a fuzzy
probability cloud, might look something like the image below:
means a surface within which we will find the electron, say 90% of the time. In section the orbital on the left, now really a fuzzy
probability cloud, might look something like the image below:
There is a shell (in yellow) where the electron is most likely to be found and another smaller shell inside this, where the electron
is also highly likely to be (also in yellow). In-between these two shells, which really correspond to the crests of waves, the
electron is less likely to be found (shown in red and corresponding to the troughs of waves). Outside the outer shell, the chance
of finding the electron slowly becomes vanishingly small and the shell is 'fuzzy'. (Note, these images are not exact plots but
merely artistic representations, though it is possible with a computer to generate exact plots from the wave function).
Which electron is which?
I have talked about 'the' electron in the hydrogen atom as if it has a definite identity, but it doesn't! Electrons come and go. In the
(otherwise empty) vacuum of space, electrons constantly appear from nothing, along with their antimatter partners, the
positron, in electron-positron pairs. When an electron and positron meet they annihilate each other again, so in the long run we
still have nothing, we just 'borrowed' some energy for a while. However, the electron that annihilates is not necessarily the
original partner of the positron, any electron will do. Often electron-positron pairs appear beside our hydrogen atom (or even
inside it?) and the positron then annihilates with the atom's electron, leaving the newly created electron to take its place. Thus,
the identity of the electron constantly changes, but since electrons with the same quantum numbers are indistinguishable, it
doesn't really matter, except that it alters the average energy of the electron slightly as it appears to jump about as it disappears
to be replaced by another electron nearby; this happens all the time!
Energy levels
Scientists often talk about the energy level of an atom (more specifically of an electron in an atom). The higher the principle
quantum number, the higher the energy of the electron and the greater is its most probable distance from the nucleus. (In this
sense atomic electrons do slightly resemble planets orbiting a central star). Thus we speak of the 300 as being larger and higher
in energy than the 200 orbital. The word 'orbital' is actually a misnomer which stems from the old idea of the electron orbiting the
nucleus as a planet orbits the Sun. However, when an electron is in a single harmonic state it does not orbit the nucleus,
according to the most commonly accepted interpretation of quantum mechanics, an electron in a single orbital does not orbit the
nucleus since it is in a stationary state (or energy eigenstate as it is called) meaning that there is no change over time. Only
when the electron is in a mixed state consisting of several harmonics superposed (rather like we added waves together when
they overlapped) can the electron exhibit any kind of orbital motion. Many scientists confuse this issue. This is a point that needs
further clarification, since the electron does have a speed when its speed is measured, but prior to the measurement it had no
definable speed since the measurement changed the electron causing it to have position and speed! Don't worry if you find this
last point hard to grasp, most scientists get it wrong too!
Technical note: Spectroscopic notation
We may talk about a 1s orbital or a 2s or 2p or 3d or 4f orbital, etc. The first number is the principle quantum number, n. The
letter designates the orbital angular momentum quantum number, l, as follows:
for l = 0, we have s
for l = 1, we have p
for l = 2, we have d
for l = 3, we have d
for l = 4, we have f
(higher numbers are designated g, h, i, ... , for l = 5, 6, 7, ... etc.).
Thus a 100 state is a 1s orbital (with m = 0). A 321 state is a 3d state (with m = 2) etc. In reality things get more complex,
especially for all the other elements whose atoms are heavier than hydrogen and which have more than one electron.
Complicating the picture we have phenomena like spin-orbit interaction which we shall not consider here. Indeed electrons
have a fourth quantum number, s, for their spin (spin or rotation - electrons have two types of 'angular momentum' one for their
rotation and another for their orbit around the nucleus. Note, that this isn't angular momentum in the ordinary sense, since
electrons do not necessarily orbit the nucleus even though they have what we call 'orbital angular momentum.' The terms spin
and orbit angular momentum are simply inherited from the study of systems that we are familiar with in the every day world.
Electrons are very different! They have l and s quantum numbers, because the properties that these describe resemble angular
momentum, but they are not angular momentum in any common sense.
Conclusion
So, the quantum world is a very strange place indeed! However, we have only scratched the surface. Coming soon, an exhibit on
the atomic nucleus, which is another very mysterious and fascinating entity!
If you would like to study atoms and quantum mechanics in depth, then consider studying with the Open University. If you have
any questions or comments then don't hesitate to contact Bot at:
BotRejectsInc@Cronodon.com
is also highly likely to be (also in yellow). In-between these two shells, which really correspond to the crests of waves, the
electron is less likely to be found (shown in red and corresponding to the troughs of waves). Outside the outer shell, the chance
of finding the electron slowly becomes vanishingly small and the shell is 'fuzzy'. (Note, these images are not exact plots but
merely artistic representations, though it is possible with a computer to generate exact plots from the wave function).
Which electron is which?
I have talked about 'the' electron in the hydrogen atom as if it has a definite identity, but it doesn't! Electrons come and go. In the
(otherwise empty) vacuum of space, electrons constantly appear from nothing, along with their antimatter partners, the
positron, in electron-positron pairs. When an electron and positron meet they annihilate each other again, so in the long run we
still have nothing, we just 'borrowed' some energy for a while. However, the electron that annihilates is not necessarily the
original partner of the positron, any electron will do. Often electron-positron pairs appear beside our hydrogen atom (or even
inside it?) and the positron then annihilates with the atom's electron, leaving the newly created electron to take its place. Thus,
the identity of the electron constantly changes, but since electrons with the same quantum numbers are indistinguishable, it
doesn't really matter, except that it alters the average energy of the electron slightly as it appears to jump about as it disappears
to be replaced by another electron nearby; this happens all the time!
Energy levels
Scientists often talk about the energy level of an atom (more specifically of an electron in an atom). The higher the principle
quantum number, the higher the energy of the electron and the greater is its most probable distance from the nucleus. (In this
sense atomic electrons do slightly resemble planets orbiting a central star). Thus we speak of the 300 as being larger and higher
in energy than the 200 orbital. The word 'orbital' is actually a misnomer which stems from the old idea of the electron orbiting the
nucleus as a planet orbits the Sun. However, when an electron is in a single harmonic state it does not orbit the nucleus,
according to the most commonly accepted interpretation of quantum mechanics, an electron in a single orbital does not orbit the
nucleus since it is in a stationary state (or energy eigenstate as it is called) meaning that there is no change over time. Only
when the electron is in a mixed state consisting of several harmonics superposed (rather like we added waves together when
they overlapped) can the electron exhibit any kind of orbital motion. Many scientists confuse this issue. This is a point that needs
further clarification, since the electron does have a speed when its speed is measured, but prior to the measurement it had no
definable speed since the measurement changed the electron causing it to have position and speed! Don't worry if you find this
last point hard to grasp, most scientists get it wrong too!
Technical note: Spectroscopic notation
We may talk about a 1s orbital or a 2s or 2p or 3d or 4f orbital, etc. The first number is the principle quantum number, n. The
letter designates the orbital angular momentum quantum number, l, as follows:
for l = 0, we have s
for l = 1, we have p
for l = 2, we have d
for l = 3, we have d
for l = 4, we have f
(higher numbers are designated g, h, i, ... , for l = 5, 6, 7, ... etc.).
Thus a 100 state is a 1s orbital (with m = 0). A 321 state is a 3d state (with m = 2) etc. In reality things get more complex,
especially for all the other elements whose atoms are heavier than hydrogen and which have more than one electron.
Complicating the picture we have phenomena like spin-orbit interaction which we shall not consider here. Indeed electrons
have a fourth quantum number, s, for their spin (spin or rotation - electrons have two types of 'angular momentum' one for their
rotation and another for their orbit around the nucleus. Note, that this isn't angular momentum in the ordinary sense, since
electrons do not necessarily orbit the nucleus even though they have what we call 'orbital angular momentum.' The terms spin
and orbit angular momentum are simply inherited from the study of systems that we are familiar with in the every day world.
Electrons are very different! They have l and s quantum numbers, because the properties that these describe resemble angular
momentum, but they are not angular momentum in any common sense.
Conclusion
So, the quantum world is a very strange place indeed! However, we have only scratched the surface. Coming soon, an exhibit on
the atomic nucleus, which is another very mysterious and fascinating entity!
If you would like to study atoms and quantum mechanics in depth, then consider studying with the Open University. If you have
any questions or comments then don't hesitate to contact Bot at:
BotRejectsInc@Cronodon.com
Comment on this article!

















